The full form of LCM is the “Least Common Multiple”. This mathematical concept is used to determine the smallest positive integer that is divisible by two or more given numbers without leaving any remainder.

LCM is a common term used in the field of mathematics. It is an abbreviation that stands for the “Least Common Multiple”. It is a commonly used concept in arithmetic, algebra, and number theory. In this article, we will delve deeper into the full form of LCM, what it means, and how it is calculated.

Calculation of LCM

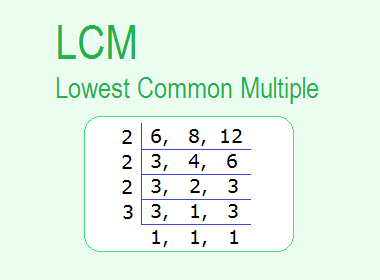
LCM is calculated by finding the product of the highest powers of all prime factors of the given numbers. For instance, let us calculate the LCM of 12 and 18.

The prime factorization of 12 is 2 x 2 x 3, while that of 18 is 2 x 3 x 3. The highest power of 2 is 2^2, while the highest power of 3 is 3^2. Thus, the LCM of 12 and 18 is 2^2 x 3^2 = 36. This means that the smallest positive integer that is divisible by both 12 and 18 is 36.

Applications of LCM

LCM is an essential concept in mathematics and has numerous applications in various fields. Here are some of the significant applications of LCM:

Fractions

LCM is used to determine the least common denominator of two or more fractions. It is the smallest common multiple of the denominators of the fractions. Once you have determined the least common denominator, you can add, subtract, or compare the fractions.

Time and Distance

LCM is also used to solve problems related to time and distance. For instance, if two cars are moving towards each other, the LCM of their speeds will give the time taken for them to meet.

Divisibility

LCM is used to determine the divisibility of two or more numbers. For example, if the LCM of two numbers is 24, then any number that is divisible by 24 is also divisible by those two numbers.

Electrical Circuits

In electrical circuits, LCM is used to determine the frequency of an alternating current. It is also used to calculate the voltage and current in a circuit.

Conclusion

In conclusion, LCM is an important mathematical concept that has numerous applications in various fields. It is the “Least Common Multiple” and is calculated by finding the product of the highest powers of all prime factors of the given numbers.

LCM is used to determine the least common denominator of fractions, solve problems related to time and distance, determine the divisibility of numbers, and in electrical circuits to calculate voltage, current, and frequency. Understanding LCM is crucial in solving various mathematical problems.